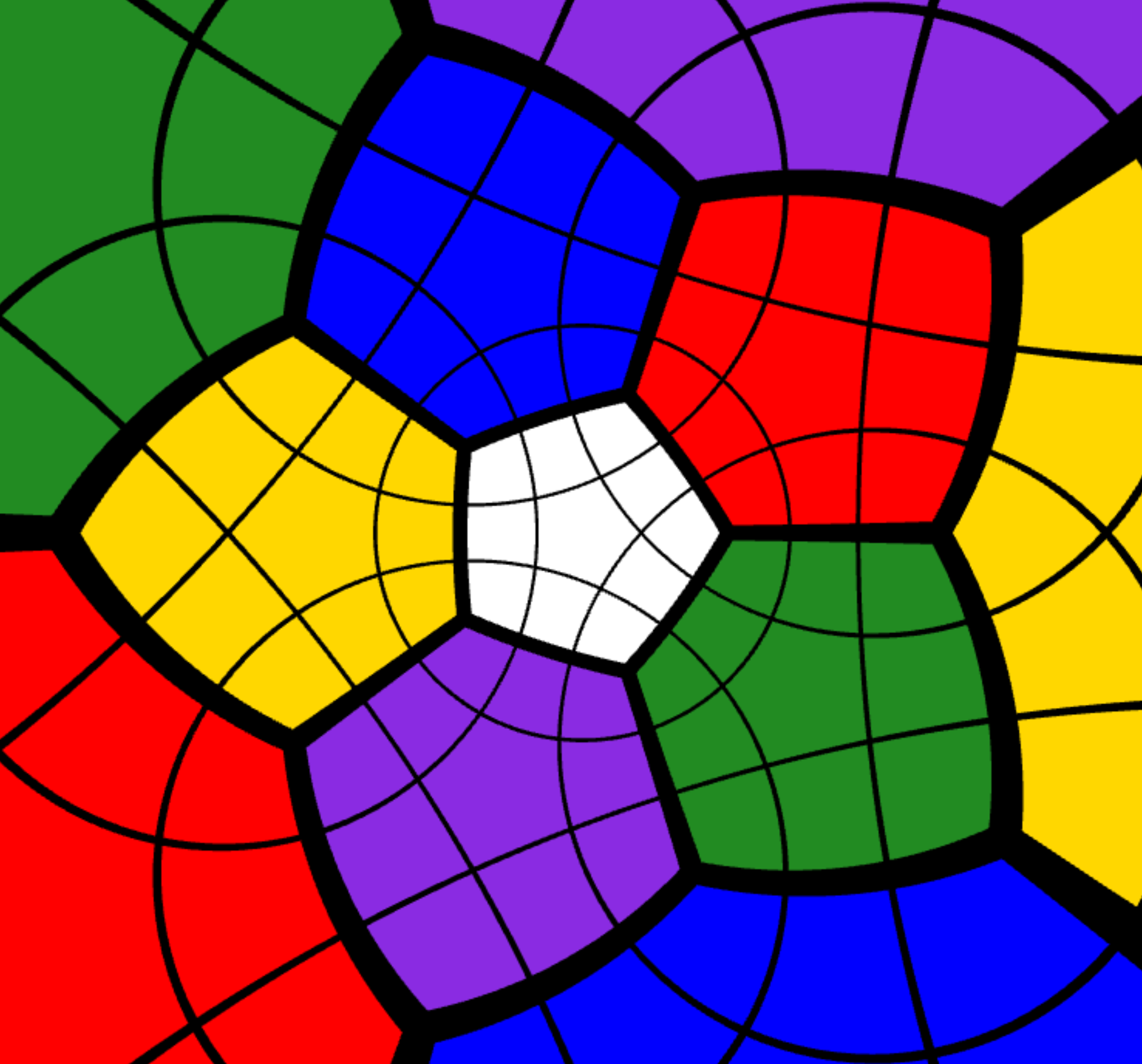
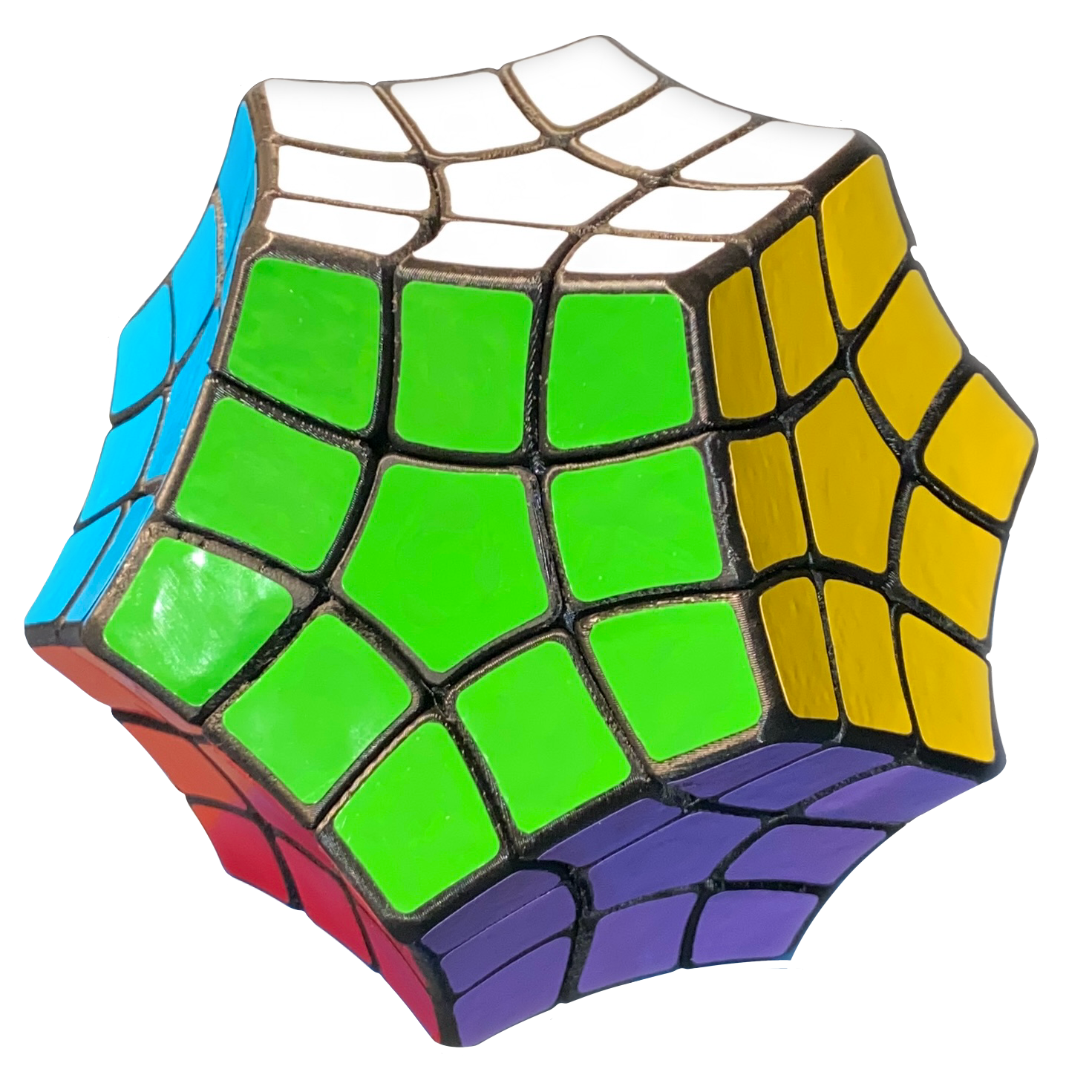
Hemimegaminx¶
The hemimegaminx is a twisty puzzle in the shape of a hemi-dodecahedron. In MagicTile, it inhabits the projective plane.
The puzzle can be constructed by identifying opposite faces on a megaminx. On this realization of the puzzle, opposite faces will turn in opposite directions. In order to be a true hemimegaminx, opposite faces should also be colored the same, so the puzzle will have 6 colors total.
Permutations¶
The 10 3c pieces of the hemimegaminx can be placed in any even permutation. Each 4c can be placed in \(3!\) orientations, except the last, which can be placed in only \(3\) orientations. The 15 2c pieces can be placed in any even permutation, and each one has \(2\) orientations, except the last, whose orientation is determined by the other pieces. Thus, the number of permutations of the puzzle is \(\left[\frac{10!}{2} \cdot 3!^{9} \cdot 3\right] \cdot \left[\frac{15!}{2} \cdot 2^{14}\right] \approx 5.87 \cdot 10^{29}.\)
Physical version¶
In 2019, after several prototypes, Oskar van Deventer built the first working hemimegaminx. In 2024, Akkei created another physical hemimegaminx and did the first known timed solve on it.